Last Updated on June 24, 2022 by Laura Turner
An awesome trick for remembering metric prefixes and converting between units in the metric system
Preparing for the MCAT requires a tremendous amount of memorization. By the time you sit down to take the exam your head will be full of facts and equations. Anything you can do to save space on your internal hard drive (aka your brain) will be a huge help on test day. If you’ve been trying to memorize the conversion factors for milliliters to liters or meters to kilometers, this trick will change your life.
But first… a little review of scientific notation.
Scientific notation is useful when we want to express very large or very small quantities. Numbers in scientific notation have the form N x 10m where 1 <N < 10 and m is an integer (a whole number).
When a number in scientific notation has a positive exponent, it indicates that the base (which is always 10) is multiplied by itself the number of times written in the exponent. 4 x 10m is 4 x 10 multiplied by 10 m times. For example, 4 times 102= 4 x 10 x 10 = 400 and 4 x 105= 4 x 10 x 10 x 10 x 10 x 10 = 40,000.
Numbers between 0 and 1 have a negative exponent, which indicates the number of times you divide by 10. For example, 4 x 10-2=4/(10 x 10)= 0.04 and 4 x 10-6= 4/(10 x 10 x 10 x 10 x 10 x 10) = 0.000004.
Sound familiar? Good.
One of the many benefits of using scientific notation is that it makes it easy to convert quantities to different units in the metric system. For example, a question will provide a quantity expressed in meters, but the appropriate formula to answer the question will require the quantity to be expressed in microns.
Because the metric system, like our number system, uses base 10, moving between units corresponds to increasing or decreasing the value of the exponent. The only real trick is keeping track of how much the exponent should change.
Medical students love mnemonics, and one (of many) that can be used to memorize the common prefixes in the metric system is
King Henry Died (gram /meter/ liter) drinking Chocolate Milk – Move 3, ’Nother 3.
The first letter of each word recalls kilo-, hecto-, deka-, (gram/meter/ liter), deci-, centi-, milli-, micro-, and nano-.
Because there are two “d” prefixes, upper and lower case are used to distinguish between deka- (“D”) and deci-(“d”). The entry (gram/ meter/ liter) refers to the type of measurement you are trying to convert. Typically this will be meters, liters or grams, but this method could also be used to convert other units such as joules to kilojoules.
Here’s a table that shows the most commonly used metric prefixes and the quantity of each measure that corresponds to 1 meter, liter or gram. For example, 1 meter is equal to .001 kilometers and 1,000 millimeters. One million nanograms is equal to 1 gram.
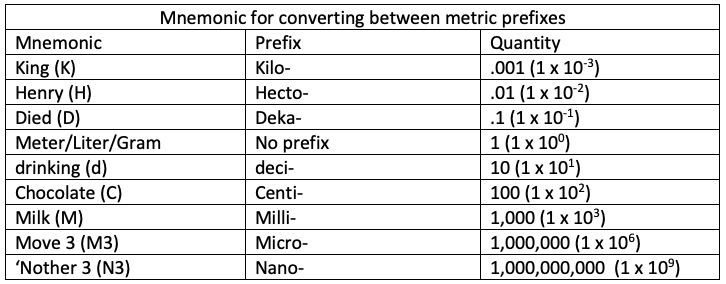
It is always worth the time to jot down the mnemonic and use it to ensure the unit conversion has been done correctly. After a bit of practice, you will only need to write the first letter of each prefix and the value of the exponent (not a full table) to jog your memory. This will be much faster and more accurate than trying to recall, for example, how many milliliters are in a 5.239 liters.
To convert from a larger to a smaller unit (from grams to milligrams, for example), add one to the exponent for each prefix you pass as you move along the list. To convert from a smaller to a larger unit (from millimeters to kilometers, for example), subtract one from the exponent for each prefix you pass as you move along the list. The exception to this is converting to the micro- and nano- prefixes, which require adding or subtracting 3 (hence the 3 in the mnemonic) from the exponent reflecting a 103 rather than 101 change in scale.
Here’s an example: A problem requires you to convert 0.3 L to milliliters. First, write “3 x 10-1 “ (which is 0.3 expressed in scientific notation) next to the entry for liters. Then add 1 to the exponent for each prefix you pass as you move from the liter row to the milliliter (M) row (because the desired units is milliliters). The full table is shown in the example for instructive purposes. With practice you will only need to write the first letter of each prefix and the value of the exponent.
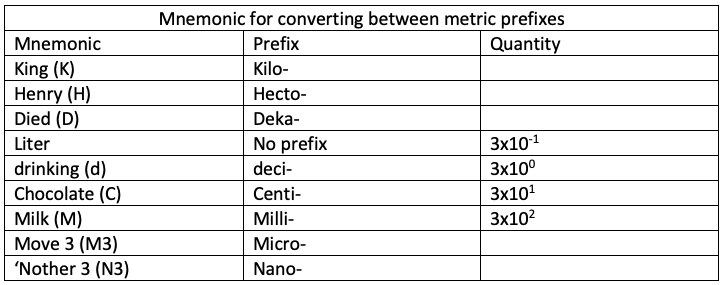
Therefore .3 liters is equal to 3 x 102 = 300 milliliters.
Another example: A problem requires you to convert 200 microns to kilometers. This time we will fill in the table with just the letter abbreviation from the mnemonic and the exponent to illustrate how quick and easy this method can be. Begin by writing “2” (to indicate the exponent in 2 x 102 which is 200 in scientific notation) in the M3 (for micrometers/microns). Then subtract 3 from the exponent to move from the M3 (for microns) to the M (for millimeters) row. Continue adding 1 to the exponent to move from M to K (for kilometers, the desired units).
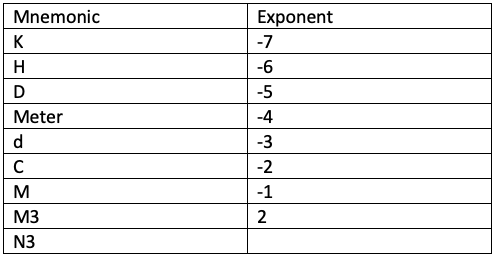
Therefore 200 microns is equal to 2 x 10-7 kilometers.
Life = changed.
- Part II – Calculating logarithms without a calculator
- Part III – Using approximation to tackle complex calculations
- Part IV – Unit conversions in eight easy steps
- Part V – The one about trigonometry
Chelsea Myers, M.Stat, is the author of the MCAT Math eBook series available at www.mcatmath.com. After a decade-long career as a research biostatistician in the Department of Ophthalmology and Visual Sciences at the University of Wisconsin – Madison, she is currently a professor of mathematics, statistics and biostatistics at Rollins College and Valencia College in Florida. She lives with her husband and three sons in the Orlando area.
I use King Henry Died by Drinking Chocolate Milk Maybe Not Pasteurized. And then it’s easy to remember micro–, nano–, and pico– are millionth, billionth, and trillionth, respectively.